The sum of squares means the sum of the squares of the given numbers. In statistics, it is the sum of the squares of the variation of a dataset. For this, we need to find the mean of the data and find the variation of each data point from the mean, square them and add them. In algebra, the sum of the square of two numbers is determined using the (a + b) 2 identity. We can also find the sum of squares of the first n natural numbers using a formula. The formula can be derived using the principle of mathematical induction. We do these basic arithmetic operations which are required in statistics and algebra. There are different techniques to find the sum of squares of given numbers.
In this article, we will discuss the different sum of squares formulas. To calculate the sum of two or more squares in an expression, the sum of squares formula is used. Also, the sum of squares formula is used to describe how well the data being modeled is represented by a model. Let us learn these along with a few solved examples in the upcoming sections for a better understanding.
| 1. | What is the Sum of Squares? |
| 2. | Sum of Squares Formula |
| 3. | Steps to Find Sum of Squares |
| 4. | Sum of Squares in Statistics |
| 5. | Sum of Squares Error |
| 6. | FAQs on Sum of Squares |
The sum of squares in statistics is a tool that is used to evaluate the dispersion of a dataset. To evaluate this, we take the sum of the square of the variation of each data point. In algebra, we find the sum of squares of two numbers using the algebraic identity of (a + b) 2 . Also, in mathematics, we find the sum of squares of n natural numbers using a specific formula which is derived using the principle of mathematical induction. Let us now discuss the formulas of finding the sum of squares in different areas of mathematics.
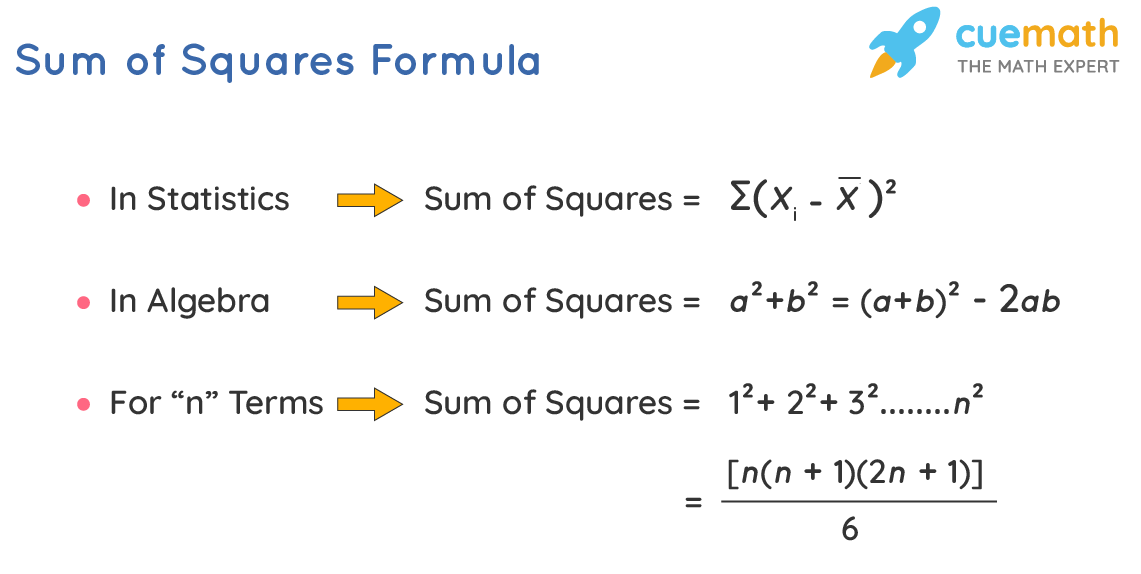
The sum of squares formula in statistics is used to describe how well the data being modeled is represented by a model. It shows the dispersion of the dataset. To calculate the sum of two or more squares in an expression, the sum of squares formula is used. Thus, a few sums of squares formulas are,
Let a and b be the two numbers. Assuming the squares of a and b are a 2 and b 2 . The sum of the squares of a and b is a 2 + b 2 . We could obtain a formula using the known algebraic identity (a+b) 2 = a 2 + b 2 + 2ab. Subtracting 2ab from both the sides we can conclude that a 2 + b 2 = (a + b) 2 - 2ab. Let a, b, c be the 3 numbers for which we are supposed to find the sum of squares. The sum of their squares is a 2 + b 2 + c 2 . Using the known algebraic identity (a+b+c) 2 = a 2 + b 2 + c 2 + 2ab + 2bc +2ca, we can evaluate that a 2 + b 2 + c 2 = (a+b+c) 2 - 2ab - 2bc - 2ca.

The total sum of squares can be calculated in statistics using the following steps:
The steps discussed above help us in finding the sum of squares in statistics. The value tells us the amount of dispersion in a dataset. It measures the variation of the data points from the mean and helps in studying the data in a better way. If the value of the sum of squares is large, then it implies that there is a high variation of the data points from the mean value. On the other hand, if the value is small, then it implies that there is a low variation of the data from its mean.
In statistics, the sum of squares error (SSE) is the difference between the observed value and the predicted value. It is also called the sum of squares residual (SSR) as it is the sum of the squares of the residual, that is, the deviation of predicted values from the actual values. The formula for the sum of squares error is given by,
SSE = ∑ n i=0 (yi - f(xi)) 2 , where yi is the i th value of the variable to be predicted, f(xi) is the predicted value, and xi is the i th value of the explanatory variable.
We can also evaluate the sum of squares error (SSE) by subtracting the sum of squares regression (SSR) from the sum of squares total (SST), that is, SSE = SST - SSR
Important Notes on Sum of Squares
☛ Related Articles:
Example 1: Using the sum of squares formula, find the value of 4 2 + 6 2 ? Solution: To find : value of 4 2 + 6 2 Given: a = 4, b = 6 Using sum of squares formula a 2 + b 2 = (a + b) 2 − 2ab, we have 4 2 + 6 2 = (4 + 6) 2 − 2(4)(6) = 100 − 2(24) = 100 − 48 = 52 Answer: The value of 4 2 + 6 2 is 52.
Example 2 : Calculate the sum of the following series 1 2 + 2 2 + 3 2 ……. 100 2 Solution: To Find: Sum of the series Using sum of squares formula for n terms, 1 2 + 2 2 + 3 2 + . + n 2 = [n(n+1)(2n+1)] / 6 Given: n =100
= [100(100+1)(2×100+1)] / 6 = (100 × 101 × 201) / 6 = 338350 Answer: The sum of the given series is 338350.
Example 3: A dataset has points 4, 5, 6, 12, 9, 10. Find the sum of squares for the given data. Solution: We have 6 data points and their sum is 4 + 5 + 6 + 12 + 9 + 10 = 46. The mean of the given data is given by, Mean, x̄ = Sum / Number of data points = 46 / 6 = 7.67 So, the sum of squares is given by, ∑ n i=0 (xi - x̄) 2 = (4 - 7.67) 2 + (5 - 7.67) 2 + (6 - 7.67) 2 + (12 - 7.67) 2 + (9 - 7.67) 2 + (10 - 7.67) 2 = (-3.67) 2 + (-2.67) 2 + (-1.67) 2 + (4.33) 2 + (1.33) 2 + (2.33) 2 = 13.4689 + 7.1289 + 2.7889 + 18.7489 + 1.7689 + 5.4289 = 49.3334 Answer: The sum of squares for the given data is 49.3334
View Answer >
Indulging in rote learning, you are likely to forget concepts. With Cuemath, you will learn visually and be surprised by the outcomes.